🎁BACK-TO-SCHOOL DEAL. Subscribe Now to get 40% OFF at only 8.49 USD/month, only valid until Sep 30th, 2024

Question

Question
LAB REPORT SHEET 34 Peptides and Proteins A. Peptides 1. Condensed structural formulas of glycine and serine Glycine Serine DH 1 0 11 1 (H₂ H₃NT CH 0 11 (-0- H₂Nt - (H₂-C-0- 2. Condensed structural formulas of dipeptides Glycylserine Serylglycine 0 0 NH3 H3 Nt - (H3 -C-NH-CH-C-0- HO-CH2-CH-C-N- (Hz-170- 0 (H₂-OH H-2-0- 3. Condensed structural formulas of the reactants and products for the hydroly- sis of serylglycine 0 tt NH3 H3N+-1H-1-0- HO-CH₂-CH-I-N-C-0- Ін 11 OP (-N1-0-Ho CH OH H₂N+- (H₂-6-0- 4. Condensed structural formula for the tripeptide 5. Abbreviations for this tripeptide 3-letter abbreviation 1-letter abbreviation

Asked By ShadowStarlight84 at

Answered By Expert

Brad

Expert · 3.2k answers · 3k people helped

Sign in to see the answer

Step 1/2

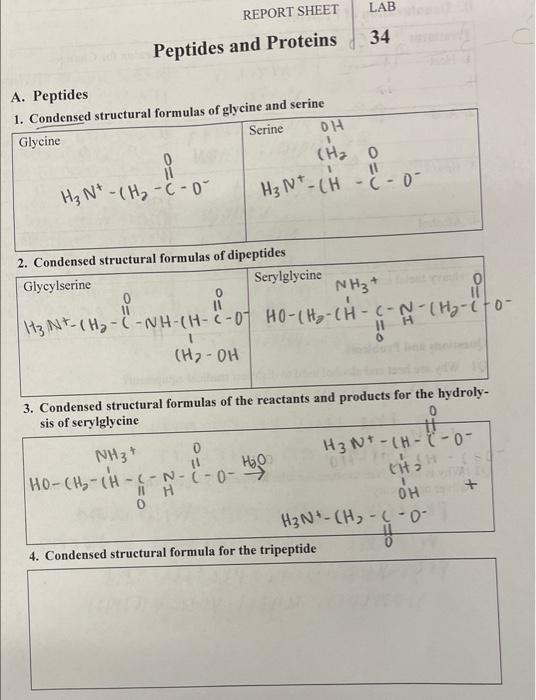
The objective of the question is to make condensed structural formula of given peptides and protien.

The expression "dense primary equation" alludes to a portrayal of a compound construction that passes on more data than a sub-atomic recipe however is less complex than a full underlying equation. Certain structural elements are written more concisely in a condensed structural formula, making it a shorthand notation for describing a molecule's atom arrangement.

Explanation:

Peptides are short chains of amino acids, which are the building blocks of proteins. Amino acids are organic compounds that contain an amino group (-NH2) and a carboxyl group (-COOH). When amino acids link together through peptide bonds, they form peptides.

Step 2/2

The condensed structure formulas of given peptides are as follows:

Serine:

Molecular Formula: `C3H7NO3`Condensed Structural Formula: ` CH₂OHCH(NH2)COOH`

`(2)~` Condensed structure formulas of dipeptides:

Glycyl serine:

Structure: ` H2N-Gly-Ser-COOH`

Serylglycine:

Structure: ` H2N-Ser-Gly-COOH ``(3)~` Condensed structure formulas of hydrolysis of Seryl-glycine:Serine: `H2N-Ser-COOH`Glycine: `H2N-Gly-COOH` `(4)~` Condensed structural formula for tripeptide.`H2​N−CH2−CO-NH−CH-CH3−CO-NH-CHCH2OH-COOH``(5)~` Abbreviation for the tripeptide is given below as:Tripeptide made out of the amino acids Alanine `(Ala)` , Valine `(Val)` , and Leucine `(Leu)` :`One-` letter code: ` AVL``Three` -letter code: `Ala-Val-Leu`

Explanation:

The hydrolysis of Serylglycine includes breaking the peptide connection between serine ( `Ser` ) and glycine ( `Gly` ), bringing about the arrangement of the singular amino acids.

Final Answer

The condensed structural formula of given compounds is shown in step `2` .

🧑‍🏫 More Questions

Which of the statements best describe spongy bone? Spongy bone is softer than compact bone. Spongy bone is composed of branches of trabeculae. Spongy bone is lighter in weight than compact bone. Spongy bone receives nutrients through osteons.

REPORT SHEET EXPERIMENT Abbreviated 32 Qualitative Analysis Scheme PART I: GROUP 1 CATIONS Record the reagent used in each step, your observations, and the equations for each precipitation reaction. Mark (+) f observed in unknown Procedure Reagent Observations G1-1 Precip G1-2 hia Dar ou Cations in group 1 unknown

QUESTION 13/17 A Windows user is locked out of her computer, and you must log into the local administrator account HelpdeskAdmin. Which would you use in the username field? WHelpdeskAdmin O // HelpdeskAdmin . HelpdeskAdmin /HelpdeskAdmin HelpdeskAdmin

REPORT SHEET Conversion Factors and Problem Solving A. Rounding Off Initial Number 143.63212 532 800 0.008 583 45 Student's 1. Correet? 2. Corrected (if needed Rounded Value (yes/no) 144 533 0.009 8.00 B. Significant Figures in Calculations B.1 Multiplication and Division Perform the following multiplication and division calculations. Give a final answer with the correct number of significant figures: 0.1 1 84 × 8.00 × 0.0345 (42.4)(15.6) 1.265 (35.56)(1.45) (4.8X0.56) B.2 Addition and Subtraction Perform the following addition and subtraction calculations. Give a final answer with the correct number of decimal places 13.45 mL+0.4552 mL 145.5 m +86.58 m+1045 m 245.625 g-80.2 g 4.62cm-0.885 cm Conversion Factors and Problem Solving 3 Area 1. Your measurements 3. Another student's measurements Length Width Area (2., 4.) Why could two students obtain different values for the calculated area of the same rectangle? B.4 Volume of a Solid 1. Shape of the solid Dimensions to measure Length Diameter (if cylinder) 2. Height Width 3. Formula for volume of solid Volume of the solid Show calculations of volume, including the units.)

Learning Objectives: For the Session 05 Pre-lab Exercise you must successfully master the following tasks in Excel: 1. Solve for a single variable using Goal Seek. 2. Solve for a system of linear equations using Matrix Algebra, Cramer's Rule, and Solver. 3. Solve for a system of non-linear equations using Solver. For this exercise, you will be given several tasks that ask you to solve for either a single variable or multiple variables. Review your lab manual before starting the pre-lab exercise. - Important - Before you begin: 1. Make sure that iterative calculations are turned on. 2. Make sure that the maximum change value is 1.0x10-15. 3. Make sure that you have the Solver add-in. Please see your lab manual for the steps needed to check the Solver add-in, turn on the iterative calculations, and to make sure that the maximum change value is set to 1.0x10-15. The pre-lab exercise and the exercise during class cannot be completed if you do not have these three things! Given the following set of linear equations: 3.2 x + 5y +7 z = 8.4 3 – 0.5 y + 6 z= 4.6 5 2 + y - 2 2= -0.7 [Equation 2.1] [Equation 2.2] [Equation 2.3] For this task, you will solve for x, y, and z using both Matrix Algebra and Cramer's Rule and answer the following questions. Question 3 What are the inverse coefficients of Equation 2.3? Give your answer to four decimal places. Inverse Coefficients: 5 x 18 1 y -2 z Question 4 Fill in the following determinants that were used for solving the system of equations with Cramer's Rule. Give your answer to two decimal places. a) D = $ b) Dx = c) Dy = G d) Dz = 4 Question 5 What are the solved values of x, y, and z? Give your answer to four decimal places. a) x = b) y = c) z = Question 6 What is the difference between using Matrix Algebra vs. Cramer's Rule? Cramer's Rule is an iterative method, whereas Matrix Algebra is not. No difference since both methods give you the same results. With Cramer's Rule, you can solve for an entire system of linear equations or for a single variable, but with Matrix Algebra you can only solve for the entire system of linear equations. With Matrix Algebra, you can solve for an entire system of linear equations or for a single variable, but with Cramer's Rule you can only solve for the entire system of linear equations. Matrix Algebra is an iterative method, whereas Cramer's Rule is not.